Dried Beans…CALCULUS. Woo…Well, its basically pretty conceptual & if you follow the ten step that follow, you’ll be in a better position to ace calculus!
Do remember that these are steps and not ways to ace calculus. So you’ll need to go step-by-step. You can move on to the next step only after the execution of the first(mathematicians are programmers).
1. Ace PreCalculus Your precalculus must be very strong if you want to move on to Calculus.
To strengthen PreCalculus, you’ll need to master:
-
- Algebra
- Trigonometry
- Geometry
(including equations & inequalities, trigonometric identities, conics, the binomial theorem, sequences & series.)
If you’re planning to take up multivariable calculus as well, you also need to build up concepts of:
-
- Vectors
- Parametric coordinates & equations
- Matrices
as a part of precalculus itself.
Now, once you are able to see functions all around you…you’re ready to go!
2. Functions are Calculus The first course I took up for Calculus was a 6 month course. My instructor took 4 months just to teach functions & their graphs! That was scary, I had a competitive exam & I had to complete the rest of it in 2 months. Surprisingly, we could do it in half the time! When the functions are with you, Calculus is with you. The rest is just application of functions. But, you need to be very good with them & especially with their graphs which help a lot in giving you an insight to the problem. You can visualize the problem & what you’re being asked for & how to get it if you take a look at the graph of the functions involved in the problem. To be a master of functions, know their nature. Their likes, dislikes & hobbies. Okay, technically you need to know their domain(they want these for the x), the range(they give these y’s) & theirbasic properties(the graph tells it all).
3.Understand the Basic Principles Yes, its now time to know what you are trying to ace…Calculus. To understand the basic principles of Calculus, you jest need to ask yourself three questions: What. Who. Why.…And the answer is the same… Mr. Newton & Mr. Leibniz gave us: On your left is what we call the derivative(represented by d/dx or y’ or f’) which gives the slope of the tangent to the function at a point. Say you have the function f(x), then the slope of f(x) at x=a would be f'(a).
%7D=frac%7Bd%7D%7Bdx%7Df(x)%7C_%7B(x=a)%7D=f'(a))
On the right is the integral of the function between two points(represented by the two lines without arrow heads) which gives the area under the curve of the function between these two points.
%7D=int_%7Ba%7D%5E%7Bb%7D%20f(x)dx=F(b)-F(a))
4. Learn to Apply The Basics One you understand the above basic physical significance of calculus(integral & differential), you should learn ow to use it to solve problems. In any calculus problem, You’ll be asked to find one of these… m(slope) or A(area) directly or inderectly. Once you know the significance of the integral & derivative, solution comes to you.
5. Understanding The First principles Lets now take up the derivative. We talked about its physical significance, but how do we compute the derivative? Well, we use something called the first principle. It directly gives us the derivative of a function & we can plug in different values of x into this derivative to get slopes of tangents at varius points.
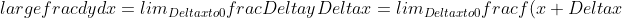-f(x)%7D%7BDelta%20x%7D)
And this is what we use to obtan the basic derivatives… You must derive all of the basic derivatives of common functions(the ones you find in anyÂ
genuine basic derivatives table). And yes, you may also derive the basic rules of differentiation(sum/product/quotient) using the first principles. You must
practice a lot of problems on differnetiation to learn the derivatives of these functions & then extend the to any possible function using the rules of differentiation.
6. Apply those Derivatives Once you are able to differentiate any function that you come accros, you would want to apply these derivatives in real time. So what can they be used for? A)You can use these for finding slopes-something you’re already familiar with. The derivative itself is the slope of the tangent at a point. What next? You can then find the equations of tangents & normals using their slopes. B)Derivatives can be used for finding maxima & minima of functions-derivatives can be used to find extremum by examining the slopes of tangents. Take a look at this curve below, you’ll find that the slope of the tangent vanishes(tangent becomes parallel to the x-axis) at points of minima & maxima.
All the x’s corresponding to these points(slope or derivative=0) are the points of extrema & the corresponding y’s, the maximum & minimum values of the function. C) The third thing derivatives help us with is determining the nature of a function or curve. We can find the intervals in which the function increases or decreses. Take a look at the image below. You’ll observe that the slope of the tangent is positive whenever the function increases while the slope is negative when the function decreases. Yeah, I know exactly how you feel!
Now the tangents 1 & 2 have a positive slope(observe that the angle they make with the x-axis < 90 degrees) & the function is increasing. Also, the tangent 3 has a negative slope(angle < 90 degrees) & the function is decreasing. Apart from the above, you can also use derivatives to sketch curves, in approximations & finding physical rates of change(which is what differential caluclus is all about).
7. Reverse the Process of Differentiation
Next, we need to reverse the differentiation process to obtain what is called the anti-derivative or indefinite integral. Indefinite, because of lack of the physical dimension to it. If the derivaive of a function f(x) is F(x), then the integral of F(x) is f(x). Thats all it means. You’ll be able to get a deeper insight if you look at
a genuine table of integrals. Knowing some of the basic integrals, you can use various methods of indefinite integration to find integrals of
almost all functions. Yes…there is a reson why I used any function in case of differnetiation & alomost any function in case of anti-differentiation. The reson is simple. You can differentiate all functions(not considering specific values) but you cannot integrate all functions. There are a few non-integrable functions.
8. Add Sense to the Anti-Derivative Its now time to go definite! This is easy, just take the anti-derivative of the function and plug in the values of the points which enclose the area.
As you see here, you may find area under a curve or the area between two curves(subtract the two shaded areas in the image).
9. Put Everything Together Put the two of them together. What do you get? Rates of Change & Areas under curves going hand in hand? Weird? But thats what calculus is all about. Thats the whole beauty of calculus. We come across a lot of weird & beautiful relation in math & Calculus gives another one. You might now wish to do some basic differential calculus to see how exactly the two process of differentiation & integration are related to each other. When you mix em’ up together, you’ll get what is called a differential equation.
10. Practice & Keep Smiling . Ok, i just reduced it to 9. Good Luck. Keep Smiling! Keep Practicing!